Navigation » List of Schools, Subjects, and Courses » Math 138 – Statistics » Quizzes » Quiz 5
No Answers We dont have answer to this question yet. If you need help with your homework send us an email  or chat with our tutors
or chat with our tutors
Quiz 5
Quiz 5
1. The Venn diagram below shows the 9 students in Ms. Tanaka’s class.
The diagram shows the memberships for the Volleyball Club and the Math Club.
Note that “Kaitlin” is outside the circles since she is not a member of either club.
One student from the class is randomly selected.
Let A denote the event “the student is in the Volleyball Club.”
Let B denote the event “the student is in the Math Club.”
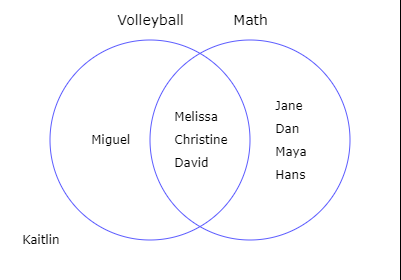
(a) Find the probabilities of the events below. Write each answer as a single fraction.
P(A) =
P(B) =
P(A and B) =
P (A or B) =
P (A) + P(B) – P (A and B) =
(b) Select the probability that is equal to P(A) + P(B) – P (A and B)
- P(A)
- P(A and B)
- P(B)
- P(A or B)
2. An urn contains 7 red and 5 black balls. Four balls are randomly drawn from the urn in succession, with replacement. That is, after each draw, the selected ball is returned to the urn. What is the probability that all balls drawn from the urn are red? Round your answer to three decimal places.
_______
3. A history class is comprised of 3 female and 5 male students. If the instructor of the class randomly chooses 6 students from the class for an oral exam, what is the probability that 2 female students and 4 male students will be selected? Round your answer to 3 decimal places.
(If necessary, consult a list of formulas.)
_________
4. A group of 118 doctors and nurses volunteered to run a health fair. Each volunteer worked one shift. The table below summarizes the data on the volunteers and their shifts.
| Morning | Afternoon | Evening | |
| Doctor | 6 | 33 | 24 |
| Nurse | 16 | 24 | 15 |
Suppose a volunteer from the health fair is chosen at random.
Answer each part. Do not round intermediate computations, and round your answers to the nearest hundredth.
What is the probability that the volunteer is a nurse or worked the morning shift?
What is the probability that the volunteer is a doctor, given that the volunteer worked the afternoon shift?
 or chat with our tutors
or chat with our tutors
