Quiz 7

1. Suppose the null hypothesis is not rejected. State the conclusion based on the results of the test.
Six years​ ago, ​12.8% of registered births were to teenage mothers. A sociologist believes that the percentage has  increased since then.
Which of the following is the correct​ conclusion?

1. There  is not sufficient evidence to conclude that the percentage of teenage mothers has remained the same.
2. There  is sufficient evidence to conclude that the percentage of teenage mothers has remained the same.
3. There is not  sufficient evidence to conclude that the percentage of teenage mothers has increased.
4. There  sufficient evidence to conclude that the percentage of teenage mothers has increased.

2.  Fill in the blank to complete the statement.
If we do not reject the null hypothesis when the statement in the alternative hypothesis is​ true, we have made a Type​ _______ error.

3. Fill in the blanks to complete the statement.
The​ _______ _______ is a statement  of no change, no effect or no difference

4. Explain what a​ P-value is. What is the criterion for rejecting the null hypothesis using the​ P-value approach?
Explain what a​ P-value is. Choose the correct answer below.

1. A​ P-value is the number of standard deviations that the observed proportion is from the proportion stated in the null hypothesis.
2. A​ P-value is the probability of observing a sample statistic as extreme or more extreme than the one observed under the assumption that the statement in the null hypothesis is true. 
3. A​ P-value is the value used to designate the area  in either the​ left- or​ right-tail of the normal curve.
4. What is the criterion for rejecting the null hypothesis using the​ P-value approach? 

5. The null and alternative hypotheses are given. Determine whether the hypothesis test is​ left-tailed, right-tailed, or​ two-tailed. What parameter is being​ tested?

Is the hypothesis test​ left-tailed, right-tailed, or​ two-tailed?

1. Left​-tailed test
2. Two​-tailed test
3. Right​-tailed test

What parameter is being​ tested?

1. Population proportion
2. Population standard deviation
3. Population mean

6. Suppose the null hypothesis is rejected. State the conclusion based on the results of the test.
Three years​ ago, the mean price of a​ single-family home was ​$243,788.  A real estate broker believes that the mean price has increased since then.
Which of the following is the correct​ conclusion?

1. There  is not sufficient evidence to conclude that the mean price of a​ single-family home has not changed. 
2. There is not sufficient evidence to conclude that the mean price of a​ single-family home has increased.
3. There is sufficient evidence to conclude that the mean price of a​ single-family home has not changed.
4. There is sufficient evidence to conclude that the mean price of a​ single-family home has increased.

7. The null and alternative hypotheses are given. Determine whether the hypothesis test is​ left-tailed, right-tailed, or​ two-tailed. What parameter is being​ tested?

$$\begin{gathered} H_0: \mu = 2 \\ {H}_1: \mu \ne 2 \end{gathered}$$

Is the hypothesis test​ left-tailed, right-tailed, or​ two-tailed?

1. Left​-tailed test
2. Two​-tailed test
3. Right​-tailed test

What parameter is being​ tested?

1. Population proportion
2. Population standard deviation
3. Population mean

8.  This past​ year, an organization conducted a survey and​ asked, “What do you think is the ideal number of children for a family to​ have?” The results of the survey are shown in the table. Complete parts​ (a) through​ (e).

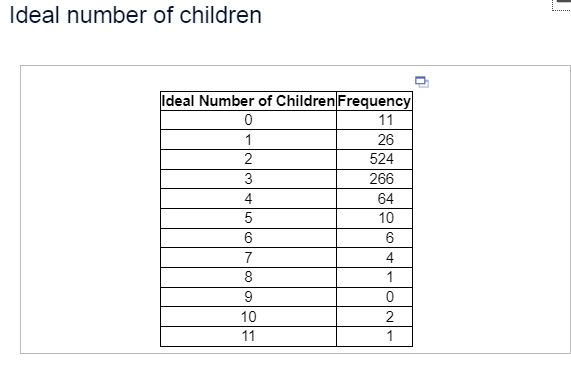
Comment on the shape of the distribution. Choose the correct answer below.

1. The distribution is symmetric.
2. The distribution is uniform.
3. The distribution is skewed left.
4. The distribution is skewed right.

(b) What is the mode ideal number of​ children? Select the correct choice​ and, if​ necessary, fill in the answer box to complete your answer.

1. The mode ideal number of children​ is _____​(Use a comma to separate answers as​ needed.)
2. There is no mode.

​(c) Determine the mean number of children.

The mean number of children is _____ (Round to two decimal places as​ needed.)

Determine the standard deviation number of children.

The standard deviation number of children is ____ ​(Round to two decimal places as​ needed.)

​(d) Explain why a large sample size is needed to perform any inference regarding this population. Choose the correct answer below.

1. The standard deviation is less than the mode.
2. The data are symmetric.
3. The mean number is greater than the standard deviation.
4. The data are clearly skewed to the right.

9.  For students who first enrolled in two year public institutions in a recent​ semester, the proportion who earned a​ bachelor’s degree within six years was 0.388. The president of a certain college believes that the proportion of students who enroll in her institution have a  completion rate.
​(a) Determine the null and alternative hypotheses.
​(b) Explain what it would mean to make a Type I error.
​(c) Explain what it would mean to make a Type II error.
​

(a) State the Hypotheses

$$\begin{gathered} H_0: \\ {H}_1: \end{gathered}$$

(b) Which of the following is a Type I​ error?

1. The president fails to reject the hypothesis that the proportion of students who earn a​ bachelor’s degree within six years is ​0.388, ​when, in​ fact, the proportion is 0.388.
2. The president rejects the hypothesis that the proportion of students who earn a​ bachelor’s degree within six years is ​0.388, ​when, in​ fact, the proportion is  greater than 0.388.
3. The president rejects the hypothesis that the proportion of students who earn a​ bachelor’s degree within six years is 0.388​, ​when, in​ fact, the proportion is 0.388.
4. The president fails to reject the hypothesis that the proportion of students who earn a​ bachelor’s degree within six years is 0.388​, ​when, in​ fact, the proportion is greater than 0.388.

(c) Which of the following is a Type II​ error?

1. The president fails to reject the hypothesis that the proportion of students who earn a​ bachelor’s degree within six years is 0.388​, ​when, in​ fact, the proportion is 03.88.
2. The president rejects the hypothesis that the proportion of students who earn a​ bachelor’s degree within six years is 0.388 ​, ​when, in​ fact, the proportion is  greater than 0.388.
3. The president fails to reject the hypothesis that the proportion of students who earn a​ bachelor’s degree within six years is 0.388​, ​when, in​ fact, the proportion is greater than 0.388.
4. The president rejects the hypothesis that the proportion of students who earn a​ bachelor’s degree within six years is 0.388​, ​when, in​ fact, the proportion is 0.388.

10. If a hypothesis is tested at the

0.05 level of​ significance, what is the probability of making a type I​ error?
Choose the correct answer below.

1. The probability of making a type I error is 0.05.
2. The probability of making a type I error is 0.5.
3. The probability of making a type I error is 0.95.
4. There is insufficient information to determine the probability of a type I error.

11. Three years​ ago, the mean price of an existing​ single-family home was ​$243,713. A real estate broker believes that existing home prices in her neighborhood are higher.
​(a) Determine the null and alternative hypotheses.
​(b) Explain what it would mean to make a Type I error.
​(c) Explain what it would mean to make a Type II error.
​

(a) State the hypotheses.
​

(b) Which of the following is a Type I​ error?

1. The broker fails to reject the hypothesis that the mean price is ​$243,713​, when the true mean price is greater than ​$243,713
2. The broker rejects the hypothesis that the mean price is ​$243,713​, when the true mean price is  greater than ​$243,713
3. The broker fails to reject the hypothesis that the mean price is ​$243,713​, when it is the true mean cost.
4. The broker rejects the hypothesis that the mean price is ​$243,713​, when it is the true mean cost.

(c) Which of the following is a Type II​ error?

1. The broker fails to reject the hypothesis that the mean price is ​$243,713​, when the true mean price is  greater than ​$243,713
2. The broker fails to reject the hypothesis that the mean price is ​$243,713​, when it is the true mean cost.
3. The broker rejects the hypothesis that the mean price is ​$243,713​, when the true mean price is greater than ​$243,713
4. The broker rejects the hypothesis that the mean price is ​$243,713​, when it is the true mean cost.

12.  Explain what it means to make a Type II error.
Choose the correct answer below.

1. Fail to reject the null hypothesis and the alternative is true.
2. Reject the null hypothesis and the null is true.
3. Reject the null hypothesis and the alternative is true.
4. Fail to reject the null hypothesis and the null is true.