Section 2.8 Homework

1.  A function f is one-to-one if different inputs produce ______  outputs. You can tell from the graph that a function is one-to-one by using the _____ test

2.  A function f has the following verbal description: “Multiply by 7, add 4, and then take the third power of the result.”

(b) Find algebraic formulas that express f and f⁻¹ in terms of the input x. 

3.  A graph of a function f is given. 

Does f have an inverse? 

1. No
2. Yes

4.  True or false?

(a) If f has an inverse, then f⁻¹(x) is always the same as

(b) If f has an inverse, then f⁻¹(f(x)) = x.

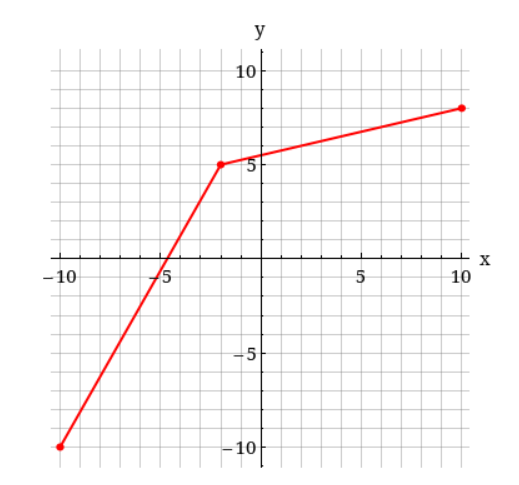
5.  A graph of a function f is given. 

Determine whether f is one-to-one. 

1. Yes, f is one-to-one.
2. No, f is not one-to-one.   

6. A graph of a function f is given. 

Determine whether f is one-to-one. 

1. Yes, f is one-to-one.
2. No, f is not one-to-one.   

7. A graph of a function f is given. 

Determine whether f is one-to-one.

1. Yes, f is one-to-one.
2. No, f is not one-to-one.    

8. Determine whether the function is one-to-one. 

$f\left(x\right) = \sqrt{7x}$

1. is one-to-one
2. is not one-to-one    

9.  Determine whether the function is one-to-one.

1. is one-to-one
2. is not one-to-one    

10. Determine whether the function is one-to-one. f(x) = x⁴ + 9

1. is one-to-one
2. is not one-to-one  

11. Assume that f is a one-to-one function.

(a) If f(4) = 9, find f ⁻¹(9).

(b) If f ⁻¹(8) = −1, find f(−1).

12. If g(x) = x² + 4x with x ≥ −2, find g⁻¹(21).

$\frac{1}{x+8}$

13. Use the Inverse Function Property to determine whether f and g are inverses of each other.

1. f and g are inverses of each other.
2. f and g are not inverses of each other. 

14.  Use the Inverse Function Property to determine whether f and g are inverses of each other. 

$f\left(x\right) = \frac{1}{x–12}, x\ne 12; g\left(x\right) = \frac{1}{x}+12$

1. f and g are inverses of each other.
2. f and g are not inverses of each other.  

15.  Find the inverse function of f. f(x) = 2 − 3x

$f^{–1}\left(x\right) =$

16. Find the inverse function of f. 

17.  Find the inverse function of f.

f(x) = 

$\frac{1}{x+8}$

 

 

18. Find the inverse function of f. 

 

$f\left(x\right)=\frac{x+8}{x–8}$

 

$f^{–1}\left(x\right)$

 

 

 

19. Find the inverse function of f. 

 

$f\left(x\right)=\frac{9x}{x–7}$

 

$f^{–1}\left(x\right) =$

 

 

20.  Find the inverse function of f. 

 

$f\left(x\right) = \frac{4x+2}{1–3x}$

$f^{–1}\left(x\right) =$

 

 

21. Find the inverse function of f. 

 

$f\left(x\right) = \frac{5–x^3}{6}$

 

$f^{–1}\left(x\right)$

 

 

22.Find the inverse function of f.

f(x) =

$={\left(x^7–6\right)}^9$

 

 

$f^{–1}\left(x\right) =$

 

 

23.  A function f is given. f(x) = 4x − 2

 

(a) Sketch the graph of f.

 

(b) Use the graph of f to sketch the graph of f⁻¹.

 

(c) Find  f⁻¹.

 

 

24.  A function f is given. f(x) = 9 − x²,    x ≥ 0

 

(a) Sketch the graph of f.

 

(b) Use the graph of f to sketch the graph of f⁻¹.

 

(c) Find f⁻¹.

 

 

25  A graphing calculator is recommended.

A one-to-one function is given.

f(x) =

$3–\frac{1}{3}x$

 

(a) Find the inverse of the function.  

$f^{–1}\left(x\right) =$

 

(b) Graph both the function and its inverse on the same screen to verify that the graphs are reflections of each other in the line y = x.

 

 

26.  A graphing calculator is recommended.

A one-to-one function is given.g(x) = 

$\sqrt{x+7}$

 

(a) Find the inverse of the function. 

 

$f^{–1}\left(x\right) =$

 

(b) Graph both the function and its inverse on the same screen to verify that the graphs are reflections of each other in the line y = x.

 

 

 

 

27.  Use the graph of f to sketch the graph of f ⁻¹. 

 

 

Graph f ⁻¹ using closed endpoints for each segment. 

 

 

 

28.  Marcello’s Pizza charges a base price of $13 for a large pizza plus $1.50 for each additional topping.

(a) Find a function f that models the price of a pizza with n toppings.

f(n) =

 

(b) Find the inverse of the function f.

$f^{–1}\left(p\right) =$

 

 

What does f⁻¹represent? 

 

1. f ⁻¹ represents the number of toppings on a pizza that costs p dollar
2. f ⁻¹ represents the cost of n toppings on a pizza.   
3. f ⁻¹ represents the number of pizzas with toppings that cost p dollars.
4. f ⁻¹ represents the cost of a pizza with n toppings.
5. f ⁻¹ represents the number of toppings on a pizza that costs 2/3(p) dollars.

 

29.  The relationship between the Fahrenheit (F) and Celsius (C) scales is given by 

 

$$\begin{gathered} F=g\left(C\right) = \frac{9}{5}C+32 \\ \left(a\right) Find g^{–1} \\ {g}^{–1}\left(F\right) = \end{gathered}$$

 

 

What does g⁻¹ represent? 

1. g⁻¹ represents the Fahrenheit temperature that corresponds to a Celsius temperature.
2. g⁻¹ represents the difference in degrees between Celsius and Fahrenheit.
3. g⁻¹ represents the Celsius temperature that corresponds to a Fahrenheit temperature.
4. g⁻¹ represents the Celsius temperature at time F.
5. g⁻¹ represents the Fahrenheit temperature at time F.

 

 

(b) Find g⁻¹(95).  g⁻¹(95) =

 

What does your answer represent?

1. It represents the Fahrenheit temperature that corresponds to 95° Celsius.
2. It represents the difference in temperature between 95° Fahrenheit and its Celsius equivalent.
3. It represents the Celsius temperature that corresponds to 95° Fahrenheit.
4. It represents the Celsius temperature after 95 minutes.

It represents the Fahrenheit temperature after 95 minutes.